Answer:
v’= 279.66 m / s
Step-by-step explanation:
We work this exercise using the conservation of the moment. For this we define the system formed by the two blocks, therefore the forces during the collision are internal of the action and reaction type.
Initial instant. Before the crash
p₀ = m v₀ + 0
Final moment. After the crash
p_f = m v + M v ’
how the tidal wave is preserved
p₀ = p_f
m v₀ = m v + M v ’
v =

let's calculate
v ’=
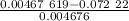
v ’=
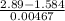
v ’= 279.66 m / s