Question

For the given table of values for a polynomial function, where must the zeros of the function lie?

A. between 3.0 and 3.5 and between 4.0 and 4.5
B. between 3.5 and 4.0 and between 4.0 and 4.5
C. between 4.0 and 4.5 and between 4.5 and 5.0
D. between 3 .5 and 4.0 and between 4.0 and 4.5
E. between 3 .5 and 4.0 and between 5.0 and 5.5

Answer 1

between 3.0 and 3.5 and between 4.0 and 4.5

Explanation:

Plato/Edmentum

Answer 2

A. Between 3.0 and 3.5 and between 4.0 and 4.5

Explanation:

The zeroes of a function occur whenever a value of x returns zero. To predict where the zeroes lie, determine the interval(s) where the function crosses the x-axis. This occurs when either
`f(x)` goes from a negative value to a positive value or vice versa.

From
`x=3.0` and
`x=3.5`, the y-values go from 4.0 (positive) to -0.2 (negative), respectively. Therefore, there must be a zero in this interval.

From
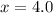`x=4.0` and
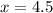`x=4.5`, the y-values go from -0.8 (negative) to 0.1 (positive), respectively. Therefore, there must also be a zero in this interval.

Thus, the zeros of this function occur between 3.0 and 3.5 and between 4.0 and 4.5, leading to answer choice A.