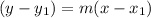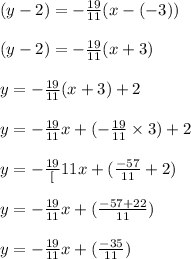Question

Find the equation of the line that passes through (–3, 2) and the intersection of the lines x–2y=0 and 3x+y+5=0.

I need the intersection point of the lines and the equation of the line.

Answer 1

`Point \ of \ intersection = ((-10)/(7) , (-5)/(7))\\\\Equation \ of \ line : y = -(19)/(11)x - (35)/(11)`

Explanation:

Find intersection of the given lines :

x - 2y = 0 => x = 2y ----------- ( 1 )

3x + y = - 5 -------------------- ( 2 )

Substitute ( 1 ) in ( 2 ) :

3x + y = - 5

3 ( 2y ) + y = - 5

6y + y = - 5

7y = - 5

`y = -(5)/(7)`

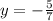
Substitute y in ( 1 ) :

x = 2y

`x = 2 * (-5)/(7) = - (10)/(7)`

`Therefore , \ point \ of \ intersection\ is ( -(10)/(7), -(5)/(7) )`

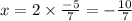
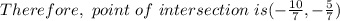
To find the equation of the line passing through ( - 3, 2) and point of intersection :

Standard equation of a line : y = mx + b , where m is the slope, b is the y intercept.

So step 1 : Find slope , m:

`slope, m = (y_2 - y_1)/(x_2 - x_1)`
`[ \ where \ (x_1, y_ 1 ) = ( -3, 2 ) \ and \ (x_2, y_ 2 ) = ( (-10)/(7) , (-5)/(7)) \ ]`

`= ((-5)/(7)-(2))/((-10)/(7) - (-3))\\\\= (-5- 14)/(-10 + 21)\\\\=(-19)/(11)\\\\=-(19)/(11)`

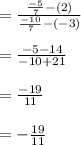
Step 2 : Equation of the line :

`(y - y _1) = m (x - x_1)\\`

`(y - 2 ) = -(19)/(11)(x -( -3))\\\\(y - 2 ) = -(19)/(11) (x+ 3)\\\\y = -(19)/(11) (x+ 3) + 2\\\\ y = -(19)/(11)x +(-(19)/(11) * 3) + 2\\\\y= - \frac{19}[11}x +((-57)/(11) + 2)\\\\y= - (19)/(11)x +((-57+ 22)/(11))\\\\y= - (19)/(11)x +((-35)/(11))\\\\`

Answer 2

`(19)/(7)`x+
`(11)/(7)`y+5=0

Explanation:

the intersection of x-2y=0 and 3x+y+5 is (
`(-10)/(7)`;
`(-5)/(7)`)

=> the line :
`(19)/(7)`x+
`(11)/(7)`y+5=0