Answer:
5000 students appeared in the examination.
Explanation:
We solve this question using Venn probabilities.
I am going to say that:
Event A: Passed in Mathematics
Event B: Passed in English.
5% failed in both subjects
This means that 100 - 5 = 95% pass in at least one, which means that
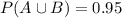
80% passed in mathematics 75% passed in english
This means that
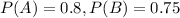
Proportion who passed in both:
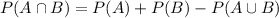
Considering the values we have for this problem
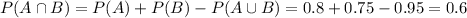
3000 of them were passed both subjects how many students appeared in the examination?
3000 is 60% of the total t. So

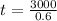

5000 students appeared in the examination.