Question

Calculate the linear acceleration of a car, the 0.220-m radius tires of which have an angular acceleration of 13.0 rad/s2. Assume no slippage and give your answer in m/s2. m/s2 (b) How many revolutions do the tires make in 2.50 s if they start from rest

Answer 1

a). 2.86
`m/s^2`

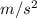
b). 6.465 revolutions

Step-by-step explanation:

Given :

a). Radius of the tires, r = 0.220 m

Angular acceleration of the tires, α = 13.0
`$rad/s^2$`

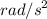
The line acceleration is defined as the rate of change of velocity with changing in direction.

The linear acceleration is equal to the product of the angular acceleration and the radius.

Therefore, linear acceleration is given by :

a = α x r

= 13 x 0.22

= 2.86
`m/s^2`

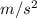
b). Given time , t = 2.5 s

The angle moved is given by :

`$\theta =(1)/(2)\alpha t^2$`

`$=(1)/(2) * 13 * (2.5)^2$`

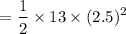
= 40.625 rad

Number of revolutions is

`$n=(\theta)/(2 \pi)$`

`$n=(4.625)/(2 \pi)$`

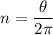
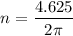
n = 6.465 revolutions