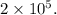Question

A steel plate of width 120mm and thickness of 20mm is bent into a circular arc radius of 10. You are required to calculate the maximum stress induced and the bending moment which will give the maximum stress. You are given that E=2*10^5​

Answer 1

Hence the magnitude of the pure moment m will be
`2* 10^5.`

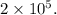
Step-by-step explanation:

• Width of steel fleet = 120 mm
• The thickness of steel fleet = 10 mm
• Let the circle of radius = 10 m

Now,

We know that,

`(M)/(I) = (E)/(R)`

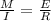
Thus,
`M =(EI)/(R)`

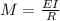
Here

R = 10000 mm

`I=(1)/(12)* 120* 10^(3)\\= 10^(4) mm^(4)`

`E=2* 10^(5)n/mm^(2)\\\\E=2* 10^(5)n/mm^(2)\\\\M={(2* 10^(5)* 10^(4))/{10000}}\\\\M=2* 10^(5)`

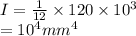
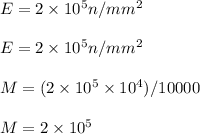
Hence, the magnitude of the pure moment m will be
`2* 10^5.`