Question

I NEED THIS ASAP PLEASE

A ball is dropped from the height of 20 feet. the ball rebounds to 80% of his previous height. Let n represent the number of bounces of the ball.
The height of the ball after each bounce can be modeled by
A. H=20(1.80)^n

B. H=20(.80)n

C. H=20(.80)^n

D. H=20(.20)^n

Answer 1

Explanation:

This is modeled after an exponential function which, at its simplest, is

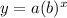`y=a(b)^x` where, for us and in this particular situation, y is the final height, a is the initial height, b is the rate of growth or decline, and x is the number of bounces. We know the initial height is 20, but we need to find the rate of decline. Rewriting the formula to model a rate of decay or decline is

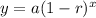`y=a(1-r)^x`, or more closely related to our circumstances:

`H=20(1-.8)^n` and simplifying that a bit:

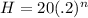`H=20(.2)^n`, choice D.