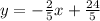Question

Find the equation of straight line passing through each of the following pairs of points

a) (2,4) and (7,2)​

Answer 1

The equation of the line is
`y = -(2)/(5)x + (24)/(5)`

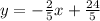
Explanation:

Equation of a line:

The equation of a line has the following format:

`y = mx + b`

In which m is the slope and b is the y-intercept.

Slope:

Having two points, the slope is given by the change in y divided by the change in x. Points (2,4) and (7,2)​, so:

Change in y: 2 - 4 = -2

Change in x: 7 - 2 = 5

Slope:
`m = (-2)/(5) = -(2)/(5)`

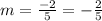
So

`y = -(2)/(5)x + b`

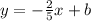
(2,4)

This means that when
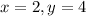`x = 2, y = 4`. So

`y = -(2)/(5)x + b`

`4 = -(2)/(5)(2) + b`

`b = 4 + (4)/(5) = (20)/(5) + (4)/(5) = (24)/(5)`

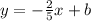
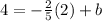
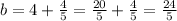
The equation of the line is
`y = -(2)/(5)x + (24)/(5)`