Given:
Principal = $54,000
Time = 9 years
Rate of compound interest = 12% per annum.
To find:
The compound interest.
Solution:
The formula for amount is:
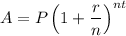
Where, P is principal, r is the rate of interest in decimal, n is the number of time interest compounded and t is the number of years.
The interest is compounded annually, so
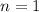 .
.
Substituting
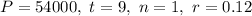 in the above formula, we get
in the above formula, we get
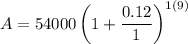
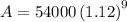
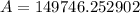
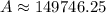
The amount after 9 years is $149746.25.
Now, the compound interest is:
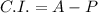
Where, C.I. is compound interest, A is amount and P is principal.

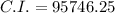
Therefore, the compound interest is $95746.25.