Question

Determine whether the given scale factor is a reduction or an

enlargement. Then scale a rectangle that has length 12 inches
and width 6 inches by 7/5. Determine the dimensions of the
scaled rectangle.

Answer 1

This is an enlargement. The dimensions of the scaled rectangle is 16.8 inches by 8.4 inches.

Step-by-step explanation:

This is a dilation.

A dilation makes a figure larger or smaller.

In this case, the scale factor is an enlargement; the figure will be larger.

The figure will be larger because the scale factor, 7/5, is larger than one (1).

If the scale factor were smaller than one (1), it would be a reduction.

In dilations, a scale factor is how much larger the image will be.

In other words, multiply the side lengths by the scale factor.

The original rectangle has a length of 12 inches and a width of 6 inches.

`12*7/5=84/5`

`6*7/5=42/5`

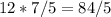
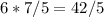
It will be more convenient to change the products, which are fractions, to decimals.

`84/5=16.8`

`42/5=8.4`

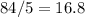
Therefore, the dimensions of the scale rectangle is 16.8 inches by 8.4 inches.

Answer 2

Answers:

• Enlargement
• The new larger rectangle is 16.8 inches by 8.4 inches

==============================================================

Step-by-step explanation:

I'm assuming 7/5 is the scale factor. If so, notice how 7/5 = 1.4 which is larger than 1.

The scale factor being larger than 1 means we have an enlargement.

The image is going to be larger compared to the preimage.

The preimage rectangle is 12 in by 6 in. Multiply each dimension by 1.4 to get 12*1.4 = 16.8 and 6*1.4 = 8.4

The image rectangle is 16.8 inches by 8.4 inches