Answer:
The weight of the planet is 29083.5 N .
Step-by-step explanation:
mass of satellite, m = 6463 kg
height of orbit, h = 4.82 x 10^5 m
period, T = 2 h
radius of planet, R = 4.29 x 10^6 m
Let the acceleration due to gravity at the planet is g.
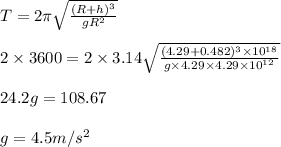
The weight of the satellite at the surface of the planet is
W = m g = 6463 x 4.5 = 29083.5 N