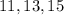Answer:
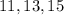
B) 6x + 16 = 82
Note: I just kept my wrong approach.
Explanation:
Let first define an odd integer.
Considering
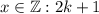 ,
,
 is an odd integer.
is an odd integer.
Now, three consecutive odd integers are
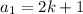 ,
,
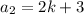 and
and
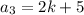
The sum of the first, two times the second and three times the third can be written as
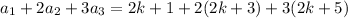
Expanding and simplifying
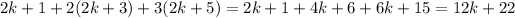
This is what I have done initially, but considering
 to be an odd integer, three consecutive integers can be written as
to be an odd integer, three consecutive integers can be written as
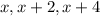 such that
such that
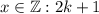
Therefore,
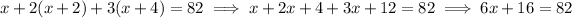
and
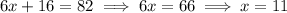
The three consecutive odd integers such that the sum of the first, two times the second and three times the third is 82 are