Solve like how you solve the system of equations. System of Equations are to find the intercept of both graphs or to find which coordinate points are solution to both equations.
We are given the equations below:
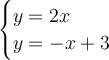
Since both equations are y-isolated and we want to find the coordinate point that both equations have or the coordinate point that is a part of two equations.
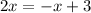
Isolate the x-term by adding both sides by x.
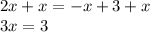
Divide both sides by 3 so we can finally get the x-isolated equation.

Next step is to find the value of y. x-term and y-term both have relations. That means when substituting x-term, we get y-term. Therefore, substitute x = 1 in any given equations. It is not necessary to substitute both, only one is fine. I will choose the first equation to solve for y-value.
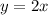
Substitute x = 1:

The value of y is 2 when x is 1. Since we want the answer to be in coordinate form or ordered pair. From (x,y) - we get (1,2).
Answer
I hope this helps! Let me know if you have any questions or doubts. Do not hestitate to ask if you've got a question. Good luck on your assignment and have a great day!