Question

I keep getting the wrong answer and have no idea what to do

Answer 1

[C] 0

General Formulas and Concepts:

Pre-Algebra

Order of Operations: BPEMDAS

1. Brackets
2. Parenthesis
3. Exponents
4. Multiplication
5. Division
6. Addition
7. Subtraction

• Left to Right

Distributive Property

Algebra I

• Terms/Coefficients
• Functions
• Function Notation

Calculus

Limits

Derivatives

Definition of a Derivative:
`\displaystyle f'(x)= \lim_(h \to 0) (f(x + h) - f(x))/(h)`

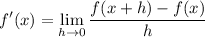
Explanation:

Step 1: Define

Identify

`\displaystyle\displaystyle g(x) = \lim_(h \to 0) (f(x + h) - f(x))/(h)`

`\displaystyle f(x) = (3)/(5x^4 + 3)`

`\displaystyle g(0)`

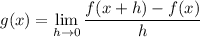
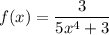
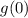
Step 2: Differentiate

1. Substitute in x [Function g(x)]:
   `\displaystyle\displaystyle g(0) = \lim_(h \to 0) (f(0 + h) - f(0))/(h)`
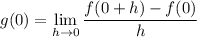
2. Substitute in function f(x) [Function g(x)]:
   `\displaystyle\displaystyle g(0) = \lim_(h \to 0) ((3)/(5(0 + h)^4 + 3) - (3)/(5(0)^4 + 3))/(h)`
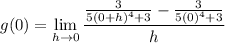
3. Simplify:
   `\displaystyle\displaystyle g(0) = \lim_(h \to 0) ((3)/(5h^4 + 3) - 1)/(h)`
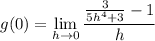
4. Rewrite:
   `\displaystyle\displaystyle g(0) = \lim_(h \to 0) (3)/(h(5h^4 + 3)) - (1)/(h)`
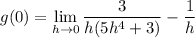
5. Rewrite:
   `\displaystyle\displaystyle g(0) = \lim_(h \to 0) (3)/(h(5h^4 + 3)) - (5h^4 + 3)/(h(5h^4 + 3))`
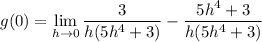
6. [Subtraction] Combine like terms:
   `\displaystyle\displaystyle g(0) = \lim_(h \to 0) (3 - 5h^4 + 3)/(h(5h^4 + 3))`
7. [Addition] Simplify:
   `\displaystyle\displaystyle g(0) = \lim_(h \to 0) (-5h^4 + 6)/(h(5h^4 + 3))`
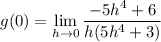
8. [Distributive Property] Distribute h:
   `\displaystyle\displaystyle g(0) = \lim_(h \to 0) (-5h^4 + 6)/(5h^5 + 3h)`
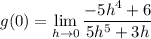
9. Evaluate limit [Power Method]:
   `\displaystyle\displaystyle g(0) = 0`

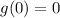
Since the bottom polynomial has a higher degree than the top polynomial, the bottom polynomial will increase faster.

∴ If the bottom is approaching a bigger value, the fraction gets smaller and smaller, approaching 0.

Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Derivatives

Book: College Calculus 10e