Answer:
5. 32I
Step-by-step explanation:
The moment of inertia of a solid sphere about its central axis is given by
I =
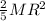 ------------------(i)
------------------(i)
Where;
M = mass of the sphere
R = radius of the sphere.
From the question;
Case 1: The aluminum sphere has a radius R and moment of inertia I.
This means that we can substitute these values of R and I into equation (i) and get;
I =
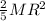 --------------(ii)
--------------(ii)
M is the mass of the aluminum sphere and is given by;
M = pV
Where;
p = density of aluminum
V = Volume of the sphere =

=> M = p(
 ) --------------------(*)
) --------------------(*)
Case 2: An aluminum sphere with a radius of 2R instead.
Let the moment of inertia in this case be I' and mass be M'
Substituting R = 2R, M = M' and I = I' into equation (i) gives
I' =
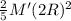 ------------------(iii)
------------------(iii)
Where;
M' = pV'
p = density of aluminum
V' = volume of the sphere =

=> M' = p(
 )
)
Rewriting gives;
M' = p(
 )
)
M' = p(
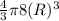 )
)
M' = 8p(
 )
)
From equation (*), this can be written as
M' = 8M
Now substitute all necessary values into equation (ii)
I' =
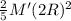
I' =
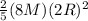
I' =
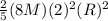
I' =
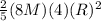
I' =
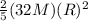
I' =
![32[(2)/(5)MR^2]](https://img.qammunity.org/2022/formulas/physics/college/e7lzunfle5z413y5mmbjtrml629nedmarg.png)
Comparing with equation (ii)
I' =
![32[I]](https://img.qammunity.org/2022/formulas/physics/college/hj37a6by3793zdt5zbj99x3zkxyrqux7fr.png)
Therefore, the moment of inertia about a central axis of a solid
aluminum sphere of radius 2R is 32I