Question

Differentiate the function. y = (3x - 1)^5(4-x^4)^5​

Answer 1

`\displaystyle y' = -5(3x-1)^4(4 - x^4)^4(15x^4 - 4x^3 - 12)`

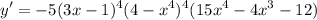
General Formulas and Concepts:

Pre-Algebra

Order of Operations: BPEMDAS

1. Brackets
2. Parenthesis
3. Exponents
4. Multiplication
5. Division
6. Addition
7. Subtraction

• Left to Right

Distributive Property

Algebra I

• Terms/Coefficients
• Factoring

Calculus

Derivatives

Derivative Notation

Derivative of a constant is 0

Basic Power Rule:

• f(x) = cxⁿ
• f’(x) = c·nxⁿ⁻¹

Derivative Rule [Product Rule]:
`\displaystyle (d)/(dx) [f(x)g(x)]=f'(x)g(x) + g'(x)f(x)`

Derivative Rule [Chain Rule]:
`\displaystyle (d)/(dx)[f(g(x))] =f'(g(x)) \cdot g'(x)`

Explanation:

Step 1: Define

Identify

y = (3x - 1)⁵(4 - x⁴)⁵

Step 2: Differentiate

1. Product Rule:
   `\displaystyle y' = (d)/(dx)[(3x - 1)^5](4 - x^4)^5 + (3x - 1)^5(d)/(dx)[(4 - x^4)^5]`
2. Chain Rule [Basic Power Rule]:
   `\displaystyle y' =[5(3x - 1)^(5-1) \cdot (d)/(dx)[3x - 1]](4 - x^4)^5 + (3x - 1)^5[5(4 - x^4)^(5-1) \cdot (d)/(dx)[(4 - x^4)]]`
3. Simplify:
   `\displaystyle y' =[5(3x - 1)^4 \cdot (d)/(dx)[3x - 1]](4 - x^4)^5 + (3x - 1)^5[5(4 - x^4)^4 \cdot (d)/(dx)[(4 - x^4)]]`
4. Basic Power Rule:
   `\displaystyle y' =[5(3x - 1)^4 \cdot 3x^(1 - 1)](4 - x^4)^5 + (3x - 1)^5[5(4 - x^4)^4 \cdot -4x^(4-1)]`
5. Simplify:
   `\displaystyle y' =[5(3x - 1)^4 \cdot 3](4 - x^4)^5 + (3x - 1)^5[5(4 - x^4)^4 \cdot -4x^3]`
6. Multiply:
   `\displaystyle y' = 15(3x - 1)^4(4 - x^4)^5 - 20x^3(3x - 1)^5(4 - x^4)^4`
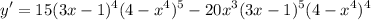
7. Factor:
   `\displaystyle y' = 5(3x-1)^4(4 - x^4)^4\bigg[ 3(4 - x^4) - 4x^3(3x - 1) \bigg]`
8. [Distributive Property] Distribute 3:
   `\displaystyle y' = 5(3x-1)^4(4 - x^4)^4\bigg[ 12 - 3x^4 - 4x^3(3x - 1) \bigg]`
9. [Distributive Property] Distribute -4x³:
   `\displaystyle y' = 5(3x-1)^4(4 - x^4)^4\bigg[ 12 - 3x^4 - 12x^4 + 4x^3 \bigg]`
10. [Brackets] Combine like terms:
    `\displaystyle y' = 5(3x-1)^4(4 - x^4)^4(-15x^4 + 4x^3 + 12)`
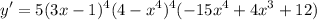
11. Factor:
    `\displaystyle y' = -5(3x-1)^4(4 - x^4)^4(15x^4 - 4x^3 - 12)`

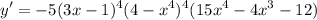
Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Derivatives

Book: College Calculus 10e