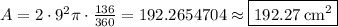Answer:
Explanation:
The area of sector with radius
 and measure
and measure
 is give by
is give by
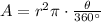 . Since vertical angles are congruent, the two shaded sectors in the figure are congruent.
. Since vertical angles are congruent, the two shaded sectors in the figure are congruent.
What we're given:
- 2 sectors of equal area
- Each sector has a radius of 9 cm
- Each sector has a measure of 136 degrees
Therefore, the area of the shaded regions (two sectors) is: