Question

Substance A decomposes at a rate proportional to the amount of A present. a) Write an equation that gives the amount A left of an initial amount A0 after time t. b) It is found that 8 lb of A will reduce to 4 lb in 4.6 hr After how long will there be only 1 lb left?

a) Choose the equation that gives A in terms of A0, t, and k, where k > 0.
b) There will be 1 lb left after 14 hr (Do not round until the final answer. Then round to the nearest whole number as needed.)

Answer 1

(a)
`A = A_0 * e^(kt)`

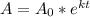
(b) There will be 1lb left after 14 hours

Explanation:

Solving (a): The equation

Since the substance decomposes at a proportional rate, then it follows the following equation

`A(t) = A_0 * e^(kt)`

Where

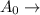`A_0 \to` Initial Amount

`k \to` rate

`t \to` time

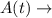`A(t) \to` Amount at time t

Solving (b):

We have:

`t = 4.6hr`

`A_0 = 8`

`A(4.6) = 4`

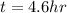
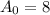
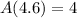
First, we calculate k using:

`A(t) = A_0 * e^(kt)`

This gives:

`A(4.6) = 8 * e^(k*4.6)`

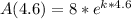
Substitute:
`A(4.6) = 4`

`4 = 8 * e^(k*4.6)`

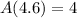
Divide both sides by 4

`0.5 = e^(k*4.6)`

Take natural logarithm of both sides

`\ln(0.5) = \ln(e^(k*4.6))`

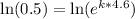
This gives:

`-0.6931 = k*4.6`

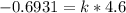
Solve for k

`k = (-0.6931)/(4.6)`

`k = -0.1507`

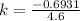
So, we have:

`A(t) = A_0 * e^(kt)`

`A(t) = 8e^(-0.1507t)`

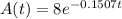
To calculate the time when 1 lb will remain, we have:

`A(t) = 1`

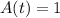
So, the equation becomes

`1= 8e^(-0.1507t)`

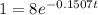
Divide both sides by 8

`0.125= e^(-0.1507t)`

Take natural logarithm of both sides

`\ln(0.125)= \ln(e^(-0.1507t))`

`-2.0794= -0.1507t`

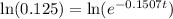
Solve for t

`t = (-2.0794)/(-0.1507)`

`t = 13.7983`

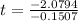
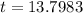
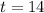`t = 14` --- approximated