Answer:
a. 54
b. 810 dollars
c. 390 dollars
d. 75 pictures
e. 561.6 dollars and 562.5 dollars
f. 38 pictures
Step-by-step explanation:
demand per week = 18 pictures
annually this demand = 18 *52 = 936
charge per unit = 60 dollars
order for 6 weeks = 6*18 = 108 quantities
cost of ordering = 45 dollars
cost of holding annually = 15 dollars
a. current average inventory
= (18*6)/2
= 54 pictures
b. current annual holding cost
(108/2)*15
= 810 dollars
c. current annual holding cost
= 936/108 * 45
= 390 dollars
d. size orders to be placed
=
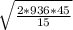
=
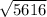
= 74.9
≈ 75 pictures have to be ordered
e. ordering holding cost per picture
936/75 * 45
= 561.6 dollars
and inventory holding cost per picture
= 75/2 * 15
=562.5 dollars
f. shop inventory per year at optimal ordering quantity
= 75/2
= 37.5
≈ 38 pictures