Question

Given circle and circle with radii of 6cm and 4cm respectively.

Compare the length of EF and GH.




The length of EF is ____the length of GH



The length of EF is___



The length of GH is ___

Answer 1

Given that :

The radius of circle A = 6 cm

The radius of circle C = 4 cm

In circle A

`\angle EAF = \theta = 140^\circ`

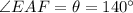
The length of arc EF =
`$2 \pi r * (\theta)/(360^\circ)$`

`$=2 * 3.14 * 6 * (140^\circ)/(360^\circ)$`

= 14.653 cm

In circle C

`\angle GCH = \theta = 140^\circ`

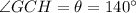
The length of arc GH =
`$2 \pi r * (\theta)/(360^\circ)$`

`$=2 * 3.14 * 4 * (140^\circ)/(360^\circ)$`

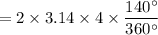
= 9.769 cm

Therefore,

The length of EF is 14.653 cm

The length of GH is 9.769 cm

The length of EF is 1.5 times the length of GH

i.e. 14.653 = 1.5 x 9.769

14.653 = 14.653

Hence proved.