Question

Please help me! i need this to pass!

Answer 1

option E, C

Explanation:

From the graph we will find the equation of g(x).

g(x) is a parabola with vertex ( h, k) = ( 0, 9)

Standard equation of parabola is , y = a (x - h)² + k

y = a (x - 0)² + 9

y = ax² + 9 ---------- ( 1 )

Now we have to find a .

To find a we will take another point through which the parabola passes .

Let it be ( 3, 0).

Substitute ( 3 , 0 ) in ( 1 ) => 0 = a (3 )² + 9

=> - 9 = 9a

=> a = - 1

Substitute a = - 1 in ( 1 ) => y = -1 x² + 9

=> y = - x² + 9

Therefore , g(x) = -x² + 9

Now using the table we will find h(x)

`h(x) = 4^(x)`

So g(x) = -x² + 9 and
`h(x) = 4^(x)`

Option A : both function increases on ( 0, ∞ ) - False

`\lim_(x \to \infty) g(x) = \lim_(x \to \infty) -x^2 + 9`

`= - \lim_(x\to \infty) x^2 + \lim_(x \to \infty) 9\\\\= - \infty + 9\\\\=- \infty`

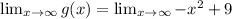
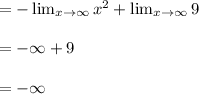
g(x) decreases on ( 0 , ∞)

`\lim_(x\to \infty) h(x) = \lim_(x \to \infty) 4^(x)`

`= \infty`

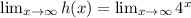
h(x) increases on ( 0, ∞)

option B : g(x) increasing on (- ∞, 0) - False

g(x) = -x² + 9

g( -2 ) = - (-2)² + 9

= - 4 + 9 = 5

g ( -5) = - ( -5)² + 9

= - 25 + 9 = - 14

As the value of x moves towards - ∞ , g(x) moves towards - ∞

Therefore g(x) decreases on (- ∞, 0)

Option C: y intercept of g(x) is greater than h(x) - True

y intercept of g(x) = ( 0 , 9 )

y intercept of h(x) = ( 0 , 1 )

Option D : h(x) is a linear function - False

Option E : g(2) < h(2) - True

g(x) = -x² + 9

g(2) = -(2)² + 9 = - 4 + 9 = 5

h(x) = 4ˣ

h(2) = 4² = 16