Answer:
Step-by-step explanation:
Here's what we know and in which dimension:
y dimension:
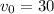 m/s
m/s
v = 0 (I'll get to that injust a second)
a = -9.8 m/s/s
The final velocity of 0 is important because that's the velocity of the ball right at the very top of its travels. If we knew how long it takes to get to that max height, we can also use that to find out how long it will take to hit the ground. Therefore, we will find the time it takes to reach its max height and pick up with the investigation of what this means after.
x dimension:
Δx = 70 m
v = ??
Velocity is our unknown.
Solving for the time in the y dimension:
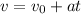 and filling in:
and filling in:
0 = 30 + (-9.8)t and
-30 = -9.8t so
t = 3.1 seconds
We know it takes 3.1 seconds to get to its max height. In order to determine how long it will take to hit the ground, just double the time. Therefore, it will take 6.2 seconds for the ball to come back to the ground, which is where the persom trying to catch the ball comes in. We will use that time in our x dimension now.
In the x dimension, the equation we need is just a glorified d = rt equation since the acceleration in this dimension is 0.
Δx = vt and
70 = v(6.2) so
v = 11.3 m/s