Question

(Please help if you can, I need this last answer done by tonight.)

Use the universal law of gravitation to solve the following problem.


Hint: mass of the Earth is = 5.97 x 1024 kg


A scientific satellite of mass 1300 kg orbits Earth 200 km above its surface. If Earth has a radius of 6378 km, what is the force of gravity acting on the scientific satellite?


a. Write out the formula for this problem.


b. Plug in the values from this problem into the formula.


c. Solve the problem, writing out each step.


d. Correct answer

Answer 1

a.

`F =G \cdot (M \cdot m)/(r^(2))`

b.

`F =6,378 * 10^(-11) * (5.97 * 10^(24) * 1,300)/(6,578^(2))`

c.

`F =6,378 * 10^(-11) * (5.97 * 10^(24) * 1,300)/(6,578^(2)) = (9.519165 * 10^(18))/(832117) \approx 1.144 * 10^(13)`

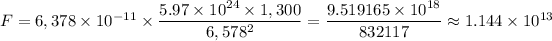
d. 1.144 × 10¹³ N

Step-by-step explanation:

The universal law of gravitation is presented as follows;

`F =G \cdot (M \cdot m)/(r^(2))`

The given mass of the scientific satellite, m = 1,300 kg

The height of the orbit of the satellite, r = 200 km above the Earth's surface

The length of the radius of the Earth, R = 6378 km

The mass of the Earth = 5.97 × 10²⁴ kg

a. The formula for the universal law of gravitation is presented as follows;

`F =G \cdot (M \cdot m)/(r^(2))`

Where;

M = The mass of the Earth = 5.97 × 10²⁴ kg

m = The mass of the satellite = 1,300 kg

r = The distance between the Earth and the satellite = R + r = 6,378 km + 200 km = 6,578 km

G = The Gravitational constant = 6.67430 × 10⁻¹¹ N·m²/kg²

b. Plugging in the values from the problem into the formula gives;

`F =6,378 * 10^(-11) * (5.97 * 10^(24) * 1,300)/(6,578^(2))`

c. Solving gives;

`F =6,378 * 10^(-11) * (5.97 * 10^(24) * 1,300)/(6,578^(2)) = (9.519165 * 10^(18))/(832117) \approx 1.144 * 10^(13)`

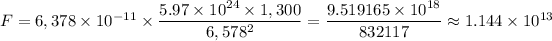
The force acting between the Earth and the satellite, F ≈ 1.144 × 10¹³ N

d. 1.144 × 10¹³ N