Question

Differentiate the function. y = (2x - 5)^2 (5 - x)?​

Answer 1

`\displaystyle y' = -(2x - 5)(6x - 25)`

General Formulas and Concepts:

Pre-Algebra

Order of Operations: BPEMDAS

1. Brackets
2. Parenthesis
3. Exponents
4. Multiplication
5. Division
6. Addition
7. Subtraction

• Left to Right

Distributive Property

Algebra I

• Terms/Coefficients
• Factoring

Calculus

Derivatives

Derivative Notation

Derivative of a constant is 0

Basic Power Rule:

• f(x) = cxⁿ
• f’(x) = c·nxⁿ⁻¹

Derivative Rule [Product Rule]:
`\displaystyle (d)/(dx) [f(x)g(x)]=f'(x)g(x) + g'(x)f(x)`

Derivative Rule [Chain Rule]:
`\displaystyle (d)/(dx)[f(g(x))] =f'(g(x)) \cdot g'(x)`

Explanation:

Step 1: Define

Identify

y = (2x - 5)²(5 - x)

Step 2: Differentiate

1. Derivative Rule [Product Rule]:
   `\displaystyle y' = (d)/(dx)[(2x - 5)^2](5 - x) + (2x - 5)^2(d)/(dx)[(5 - x)]`
2. Chain Rule [Basic Power Rule]:
   `\displaystyle y' = [2(2x - 5)^(2 - 1) \cdot (d)/(dx)[2x]](5 - x) + (2x - 5)^2(d)/(dx)[(5 - x)]`
3. Simplify:
   `\displaystyle y' = [2(2x - 5) \cdot (d)/(dx)[2x]](5 - x) + (2x - 5)^2(d)/(dx)[(5 - x)]`
4. Basic Power Rule:
   `\displaystyle y' = [2(2x - 5) \cdot 1 \cdot 2x^(1 - 1)](5 - x) + (2x - 5)^2(1 \cdot -x^(1 - 1))]`
5. Simplify:
   `\displaystyle y' = [2(2x - 5) \cdot 2](5 - x) + (2x - 5)^2(-1)`
6. Multiply:
   `\displaystyle y' = 4(2x - 5)(5 - x) - (2x - 5)^2`
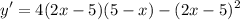
7. Factor:
   `\displaystyle y' = (2x - 5)[4(5 - x) - (2x - 5)]`
8. [Distributive Property] Distribute 4:
   `\displaystyle y' = (2x - 5)[20 - 4x - (2x - 5)]`
9. [Distributive Property] Distribute negative:
   `\displaystyle y' = (2x - 5)[20 - 4x - 2x + 5]`
10. [Subtraction] Combine like terms (x):
    `\displaystyle y' = (2x - 5)[20 - 6x + 5]`
11. [Addition] Combine like terms:
    `\displaystyle y' = (2x - 5)(25 - 6x)`
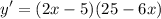
12. Factor:
    `\displaystyle y' = -(2x - 5)(6x - 25)`

Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Derivatives

Book: College Calculus 10e