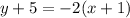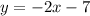Question

=
For the function f(x) = x2 – 6, find the equation of the tangent line at
X = -1.

Answer 1

`y = - 2x - 7`

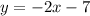
Explanation:

One way is to differentiate the function

`(d)/(dx) {x}^(2) - (d)/(dx) 6`

Use Power Rule

`2x - 0`

`2x`

P

`- 2`

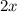
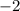
Plug in -1 so we get

That gives us the slope of the tangent line. we must find the point it passes through.

`( - 1) {}^(2) - 6 = - 5`

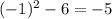
So it have a slope of -2 and passes through (-1,-5)

So we use point slope form

`y + 5 = - 2(x + 1)`

`y = - 2x - 7`