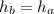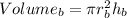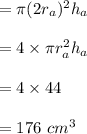Question

the volume of a cylinder is 44cm3. find the volume of another cylinder of the same height and double the base radius

Answer 1

So what you need to do is form another cylinder that has a volume of 44cm3 and then double the base size by the radius.

Answer 2

`Volume \ of \ other\ cylinder = 176 \ cm^3`

Explanation:

Let the volume of cylinder Vₐ = 44cm³

Let radius of cylinder " a " be = rₐ

Let height of cylinder " b" be = hₐ

`Volume_a = \pi r_a^2 h_a\\\\44 = \pi r_a^2 h_a`

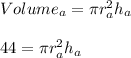
Given cylinder " b ", Radius is twice cylinder " a " , that is
`r_b = 2 r_a`

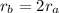
Also Height of cylinder " b " is same as cylinder " a " , that is
`h_b = h_a`

`Volume_b = \pi r_b^2 h_b`

`= \pi (2r_a)^2 h_a\\\\=4 * \pi r_a^2 h_a\\\\= 4 * 44\\\\= 176 \ cm^3`