Question

Please help solve it ​

Answer 1

2 square root 10

Explanation:

firstly you must find the hipotenus using the hipotenus formula AB is the hipotenus

(4)*2 + (2 square root 10)*2 = 40 juat press a calculator if you dont know

and then you press square root of 40 then you will get 2 square root 10 so you already prove that AB is 2 square root 10

Answer 2

Explanation:

1 to find AB

in triangle ADB

here 4 and 2
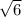`√(6)` are the legs of the right triangle so let them be a and b respectively.

AB is the hypotenuse (longest side ) and let it be x

using pythagoras theorem

a^2 + b^2 = c^2

4^2 +
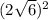`(2√(6) )^2` = AB^2

16 + 4*6 = AB^2

16 + 24 = AB^2

40 = AB^2

`√(40)` = AB

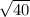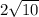`2√(10)` = AB

therefore AB =v
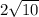`2√(10)` .Hence proved .

In triangle ADC

here 6 and
`2√(6)` are the legs of the right angle so let them be a and b respectively. AC is the hypotenuse (longest side) so let it be c

usinfg pythagoras theorem

a^2 + b^2 = c^2

6^2 +
`(2√(6) )^2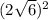` = AC^2

36 + 4*6 = AC^2

36 + 24 = AC^2

60 = AC^2

`√(60)` = AC

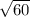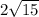`2√(15)` = AC

To prove triangle ABC is a right angled triangle

AD and BD are the legs and AB is hypotenuse

according to pythagoras theorem to be the right angle triangle the sum of square of two smaller sides of a triangle must equal to the square of hypotenuse(longest side)

let two smaller sides be a and b respectively and c be hypotenuse

using pythagoras theorem

a^2 + b^2 = c^2

4^2 +
`(2√(6) )^2` =
`(2√(10) )^2`

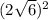16 +2^2*6 = 2^2*10

16 + 4*6 = 4*10

16 + 24 = 40

40 = 40

since both sides are equal it is proved that the triangle ABC is a right angled triangle.

Hope this helps u !!