Question

5. Two obeserves Pand Q 15 m apart observe a kite in

the same vertical plane and from the same side of
the kite. The angle of elevation of the kite from P and Q are
35° and 45°respectively. Find the height
ar the kite to the nearest metre.
(WAEC​

Answer 1

Approximately
`35\; \rm m`.

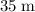Explanation:

In a right triangle, the tangent of an angle is the ratio between the length of the side opposite to this angle and the length of the side adjacent to this angle:

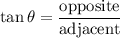`\displaystyle tan(\theta) = \frac{\text{opposite}}{\text{adjacent}}`.

Let
`x` denote the height of this kite in meters.

Refer to the diagram attached. Let
`\sf O` denote the point right below the kite in the same vertical plane as
`\sf P` and
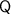`\sf Q`. Denote the kite as
`\sf K`.

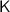The kite
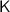`\sf K`, observer
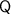`\sf Q`, and point
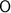`\sf O` are the three vertices of right triangle
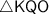`\triangle {\sf KQO}`, where
`\angle{\sf KQO} = 45^\circ`.

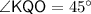In this right triangle,
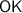`\sf OK` is the side opposite to
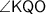`\angle{\sf KQO}`, whereas
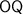`\sf OQ` is the side adjacent to
`\angle{\sf KQO}\!`.

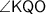Therefore:

`\displaystyle \tan(\angle{\sf KQO}) = \frac{\text{length of ${\sf OK}$}}{\text{length of ${\sf OQ}$}}`.

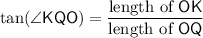• The length of
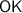  `\sf OK` is the same as the height of this kite,
  `x` meters.
• The length of
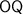  `\sf OQ` denotes the horizontal distance between the kite and observer
  `\sf Q`.

`\displaystyle \tan(45^\circ) = \frac{x}{\text{length of ${\sf OQ}$}}`.

`\text{length of ${\sf OQ}$} = x\, \tan(45^\circ)`.

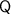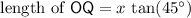Similarly, in right triangle
`\triangle {\sf KPO}`,
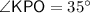`\angle{\sf KPO} = 35^\circ`. In this right triangle,
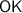`\sf OK` is the side opposite to
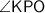`\angle{\sf KPO}`, whereas
`\sf OP` is the side adjacent to
`\angle{\sf KPO}\!`.

`\displaystyle \tan(\angle{\sf KPO}) = \frac{\text{length of ${\sf OK}$}}{\text{length of ${\sf OP}$}}`.

`\displaystyle \tan(35^\circ) = \frac{x}{\text{length of ${\sf OP}$}}`.

`\text{length of ${\sf OP}$} = x\, \tan(35^\circ)`

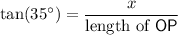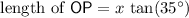
The question states that observer
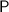`\sf P` and observer
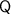`\sf Q` are on the same side of the kite. Hence, the horizontal distance between
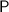`\sf P\!` and
`\sf Q\!` would be the same as the difference between:

• the horizontal distance between
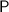  `\sf P` and the kite (same as the length of segment
  `\sf OP`,) and
• the horizontal distance between
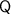  `\sf Q` and the kite (same as the length of segment
  `\sf OQ`.)

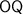In other words:

`\begin{aligned}& \text{horizontal distance between ${\sf P}$ and ${\sf Q}$} \\ &= (\text{horizontal distance between ${\sf P}$ and ${\sf K}$} \\ &\quad\quad -\text{horizontal distance between ${\sf Q}$ and ${\sf K}$})\end{aligned}`.

`\begin{aligned}& \text{length of ${\sf PQ}$} \\ &= \text{length of ${\sf OP}$} - \text{length of ${\sf OQ}$}\end{aligned}`.

`\displaystyle 15 = (x)/(\tan(35^\circ)) - (x)/(\tan(45^\circ))`.

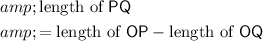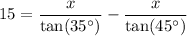Solve for
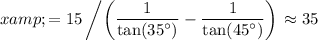`x`:

`\begin{aligned}x &= 15 \left/\left(\frac{1}{\tan({35}^\circ)} - \frac{1}{\tan({45}^\circ)}\right)\right. \approx 35\end{aligned}`.