Question

The body moves with the speed v=5t^2-2t+2. What is its speed at the moment when the acceleration is zero?​

Answer 1

1.8m/s

Explanation:

Given:

speed;

v = 5t² - 2t + 2 ---------------(i)

(i) First, find the acceleration a by differentiating the speed equation in equation (i) with respect to t as follows;

a =
`(dv)/(dt)`

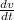
a =
`(d)/(dt) [5t^2 - 2t + 2]`

a = 10t - 2 ---------------------(ii)

(ii) Next, find the moment (time) when the acceleration is zero. This is done by substituting a = 0 into equation(ii) as follows;

0 = 10t - 2

Solve for t

10t = 2

t = 2 / 10 = 0.2

Therefore, at t = 0.2s, the acceleration is zero.

(iii) Now, find the velocity at time t = 0.2s when the acceleration is zero. This is done by substituting the value of t = 0.2s into equation (i) as follows;

v = 5(0.2)² - 2(0.2) + 2

v = 0.2 - 0.4 + 2

v = 1.8

Therefore, the speed at the moment when acceleration is zero is 1.8m/s

NB: Since the units of measurement are not given, the S.I units are used. Hence m/s for speed.