Answer:
There is a sufficient evidence to support the that machine 1 has the greater variance.
Explanation:
The question with the complete details can be found online.
Start by stating the hypotheses.
The null hypothesis states that the variance of machine 1 is less than or equal to machine 2
So, we have:
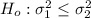
The alternate hypothesis will then be:
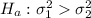
So, we have:
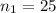
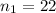
Calculate the mean of Machine 1 and 2
The mean is:
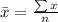
For machine 1, we have:
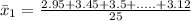
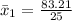
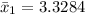
For machine 2, we have:
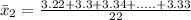
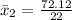
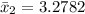
Calculate the standard deviation of both machines
The standard deviation is:
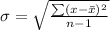
For machine 1, we have:
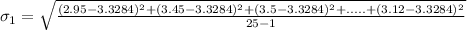
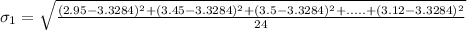
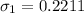
For machine 2, we have:
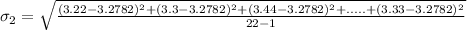

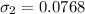
Calculate the degrees of freedom
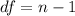
For machine 1;
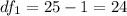
For machine 2
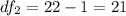
Calculate the test statistic (t)
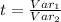
Rewrite in terms of standard deviation
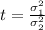
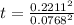
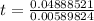
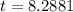
Lastly, calculate the p value.
This is the value of P(t > 8.2881) between the degrees of freedom i.e. 21 and 24
From the f distribution table
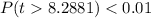
Hence, we reject the null hypothesis