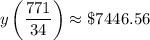Question

A company sells widgets. The amount of profit, y, made by the company, is related to the selling price of each widget, x, by the given equation. Using this equation, find out what price the widgets should be sold for, to the nearest cent, for the company to make the maximum profit.

y=-34x^2+1542x-10037

Answer 1

In order to maximize profit, the company should sell each widget at $22.68.

Explanation:

The amount of profit y made by the company for selling widgets at x price is given by the equation:

`y=-34x^2+1542x-10037`

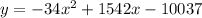
And we want to find to price for which the company should sell in order to maximize the profit.

Since our equation is a quadratic with a negative leading coefficient, its maximum will occur at the vertex point.

The vertex of a quadratic is given by the formulas:

`\displaystyle \text{Vertex}=\left(-(b)/(2a), f\left(-(b)/(2a)\right)\right)`

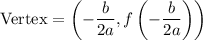
In this case, a = -34, b = 1542, and c = -10037.

Find the x-coordinate of the vertex:

`\displaystyle x=-((1542))/(2(-34))=(771)/(34)\approx \$22.68`

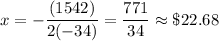
So, in order to maximize profit, the company should sell each widget at $22.68.

Extra Notes:

In order to find the maximum profit, substitute the price back into the equation:

`\displaystyle y\left((771)/(34)\right)\approx\$7446.56`