Question

What is the value of X in the figure below? In this diagram ∆ABD is similar to ∆CAD.

Answer 1

• F. 45/4

Explanation:

Corresponding sides have same ratio:

• AB/AC = BD/AD

Find AB:

• AB =
  `√(20^2-15^2) = 5√(7)`

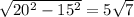
Find AD:

• AD = √15²-x²

Now the ratio:

• 5√7/15 = (20 - x)/√(15²-x²)
• √7 *√(225-x²)= 3(20 - x)
• 7(225 - x²) = 9(20 -x)²
• 7*225 - 7x² = 9(400 - 40x + x²)
• 1575 - 7x² = 3600 - 360x + 9x²
• 16x² - 360x + 2025 = 0
• (4x)² - 2*4x*45 + 45² = 0
• (4x - 45)² = 0
• 4x - 45 = 0
• 4x = 45
• x = 45/4

Correct choice is F