Question

annual survey in which readers rate their favorite cruise ship. All ships are rated on a -point scale, with higher values indicating better service. A sample of ships that carry fewer than passengers resulted in an average rating of , and a sample of ships that carry or more passengers provided an average rating of . Assume that the population standard deviation is for ships that carry fewer than passengers and for ships that carry or more passengers. Round your all answers to two decimal places.

Answer 1

This question is incomplete, the complete question is;

Condé Nast Traveler conducts an annual survey in which readers rate their favorite cruise ship. All ships are rated on a 100-point scale, with higher values indicating better service.

A sample of 37 ships that carry fewer than 500 passengers resulted in an average rating of 85.36, and a sample of 44 ships that carry 500 or more passengers provided an average rating of 81.40.

Assume that the population standard deviation is 4.55 for ships that carry fewer than 500 passengers and 3.97 for ships that carry 500 or more passengers. What is the value of the test statistic? Round your all answers to two decimal places.

Answer:

value of the test statistic is 4.13

Explanation:

Given the data in the question;

fewer than 500 passengers more than 500 passengers

n₁ = 37 n₂ = 44

x'₁ = 85.36 x'₂ = 81.40

s₁ = 4.55 s₂ = 3.97

Now, to get the test statistics, we use the following formula;

z = (x'₁ - x'₂) / √(
`(s_1^2)/(n_1) + (s_2^2)/(n_2)` )

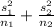we substitute

z = ( 85.36 - 81.40 ) / √(
`((4.55)^2)/(37) + ((3.97)^2)/(44)` )

z = 3.96 / √(
`(20.7025)/(37) + (15.7609)/(44)` )

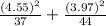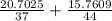z = 3.96 / √( 0.5595 + 0.3582 )

z = 3.96 / √0.9177

z = 3.96 / 0.957966596

z = 4.1337 ≈ 4.13

Therefore, value of the test statistic is 4.13