Question

What is the area in square inches of this quadrilateral

Answer 1

160 in²

Explanation:

20 * 16 / 2

if we wouldn't devide with 2, we would calculate the rectangle around the quadrilateral

Answer 2

G, 160 in^2

Explanation:

Let us start with the general equation to find the area of this quadrilateral, which is noticably a rhombus,
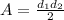`A=(d_1d_2)/(2)`, where the two d represent the two diagonals of the rhombus.

Notice that the longer diagonal consists of two 10in. portions. Let's add them up to find the total length:

`10+10=20`in.

Now let's find the smaller diagonal consisting of two 8 in. portions:

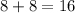`8+8=16`in.

Great! Let's plug in to solve for the area:

`A=(20*16)/(2) \\A=(320)/(2) \\A=160`

Therefore, the area of the quadrilateral is G, 160 in^2.

I hope this helps! Let me know if you have any questions :)