Question

Quadrilateral QRST is a parallelogram. Solve for x (3x+5) (9x-17)

Answer 1

Given:

Consider the below figure attached with this equation.

Quadrilateral QRST is a parallelogram.

To find:

The value of x.

Solution:

We know that the sum of two consecutive interior angles of a parallelogram is 180 degrees because they are supplementary angles.

In parallelogram QRST,

`m\angle Q+m\angle T=180^\circ`

`(3x+5)^\circ+(9x-17)^\circ=180^\circ`

`(12x-12)^\circ=180^\circ`

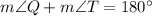
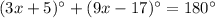
On comparing both sides, we get

`12x-12=180`

`12x=180+12`

`12x=192`

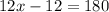
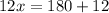
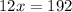
Divide both sides by 12.

`x=(192)/(12)`

`x=16`

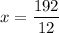
Therefore, the value of x is 16.