Question

A circle has a center at (-2, 5) and a radius of bold 3 square root of bold 2units. What is the equation of the circle in standard form?

Answer 1

Given:

The center of the circle = (-2,5)

The radius of the circle =
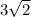`3√(2)` units.

To find:

The equation of the circle is standard form.

Solution:

The standard form of a circle is:

`(x-h)^2+(y-k)^2=r^2` ...(i)

Where, (h,k) is the center of the circle and r is the radius of the circle.

It is given that the center of the circle is (-2,5). So,
`h=-2,\ k=5`.

The radius of the circle is
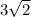`3√(2)` units. So,
`r=3√(2)`.

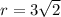Putting
`h=-2,\ k=5` and
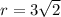`r=3√(2)` in (i), we get

`(x-(-2))^2+(y-(5))^2=(3√(2))^2`

`(x+2)^2+(y-5)^2=18`

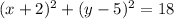
Therefore, the equation of the circle is
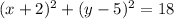`(x+2)^2+(y-5)^2=18`.