1 Answer

Sbonkosky · Answer 1 · 2022-05-21T12:20:48+0000

======================================================

Step-by-step explanation:

Plug in x = 1

f(x) = 17-x^2

f(1) = 17-1^2

f(1) = 17-1

f(1) = 16

Repeat for x = 5 to find that f(5) = -8

Now we'll use the formula below to find the average rate of change from x = a to x = b.

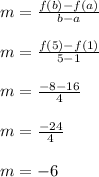
$m = (f(b)-f(a))/(b-a)\\\\m = (f(5)-f(1))/(5-1)\\\\m = (-8-16)/(4)\\\\m = (-24)/(4)\\\\m = -6\\\\$

The average rate of change is -6

The formula is basically the slope formula, more or less. So that's why I used 'm' to represent the average rate of change.

The average rate of change on the interval [1,5] is the same as finding the slope through the lines (1, 16) and (5, -8)

Please log in or register to add a comment.