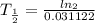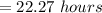Answer:
The correct answer is "22.27 hours".
Step-by-step explanation:
Given that:
Radioactive isotope activity,
= 490,000 Bq
Activity,
= 110,000 Bq
Time,
= 48 hours
As we know,
⇒
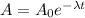
or,
⇒
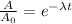
By taking "ln", we get
⇒
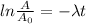
By substituting the values, we get
⇒
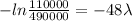
⇒
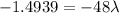
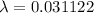
As,
⇒

then,
⇒
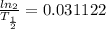
⇒