Question

What number must you add to complete the square? x^2+28x=11

Answer 1

• x²+28x+11

To complete 2ab of (a+b)²

• 2ab=28x
• 2x(b)=28x
• b=28x/2x
• b=14
• b²=196

196 must be added on both sides to get complete square as (x+14)²

Answer 2

196

Explanation:

Given equation:

`x^2+28x=11`

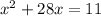
Step 1

When completing the square for an equation in the form ax²+bx+c=0, the first step is to move the constant to the right side of the equation.

This has already been done in the given equation:

`x^2+28x=11`

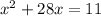
Step 2

Add the square of half the coefficient of x to both sides.

This forms a perfect square trinomial on the left side:

`\implies x^2+28x+\left((28)/(2)\right)^2=11+\left((28)/(2)\right)^2`

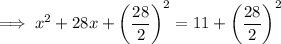
Simplify:

`\implies x^2+28x+14^2=11+14^2`

`\implies x^2+28x+196=11+196`

`\implies x^2+28x+196=207`

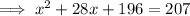
Step 3

Factor the perfect square trinomial on the left side:

`\implies (x+14)^2=207`

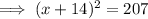
We have now completed the square and can go onto solving the equation.

Therefore, 196 must be added to both sides of the equation to complete the square.