Question

In ΔVWX, the measure of ∠X=90°, XW = 65, WV = 97, and VX = 72. What is the value of the cosine of ∠V to the nearest hundredth?

Answer 1

The cosine of ∠V is of 0.74.

Explanation:

Relations in a right triangle:

The cosine of an angle is given by the length of the adjacent side divided by the length of the hypotenuse.

XW = 65, WV = 97, and VX = 72.

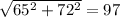`√(65^2+72^2) = 97`, and thus, this is a right triangle.

What is the value of the cosine of ∠V to the nearest hundredth?

The hypotenuse is the largest side, that is, WV = 97.

The adjacent side of angle V is VX = 72. So

`cos(B) = (72)/(97) = 0.74`

The cosine of ∠V is of 0.74.