Given:
Two expressions are
![\left[\left(-(1)/(5)\right)+\left(-(3)/(5)\right)\right]+\left((1)/(7)\right)](https://img.qammunity.org/2022/formulas/mathematics/college/fk4uenjk9fjpie1s022plahr9qyc9ht5ci.png) and
and
![\left(-(1)/(5)\right)+\left[\left(-(3)/(5)\right)+\left((1)/(7)\right)\right]](https://img.qammunity.org/2022/formulas/mathematics/college/ldpoo8zti84nqrz9htai83nurqbsam3jfg.png) .
.
To find:
The property that allows to compute
![\left[\left(-(1)/(5)\right)+\left(-(3)/(5)\right)\right]+\left((1)/(7)\right)](https://img.qammunity.org/2022/formulas/mathematics/college/fk4uenjk9fjpie1s022plahr9qyc9ht5ci.png) as
as
![\left(-(1)/(5)\right)+\left[\left(-(3)/(5)\right)+\left((1)/(7)\right)\right]](https://img.qammunity.org/2022/formulas/mathematics/college/ldpoo8zti84nqrz9htai83nurqbsam3jfg.png) .
.
Solution:
According to associative property of addition, if a, b and c are real numbers, then
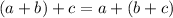
Using the associative property of addition, we get
![\left[\left(-(1)/(5)\right)+\left(-(3)/(5)\right)\right]+\left((1)/(7)\right)=\left(-(1)/(5)\right)+\left[\left(-(3)/(5)\right)+\left((1)/(7)\right)\right]](https://img.qammunity.org/2022/formulas/mathematics/college/uxjyvir9tkdsyxt13gm3zkw18hmf3jm5u4.png)
Therefore, the required property is associative property of addition.