Question

Renee has dimes and quarters in her wallet that total $1.60. She has 2 more dimes than quarters.

How many of each coin does Renee have?

Answer 1

Given:

Renee has dimes and quarters in her wallet that total $1.60.

She has 2 more dimes than quarters.

To find:

The number of each type of coins.

Solution:

Let x be the number of dimes and y be the number of quarters.

She has 2 more dimes than quarters. So,

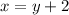`x=y+2` ...(i)

We know that,

1 dime = $0.10

1 quarter = $0.25

Renee has dimes and quarters in her wallet that total $1.60. So,

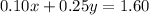`0.10x+0.25y=1.60` ...(ii)

From (i) and (ii), we get

`0.10(y+2)+0.25y=1.60`

`0.10y+0.20+0.25y=1.60`

`0.35y=1.60-0.20`

`0.35y=1.40`

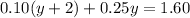
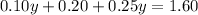
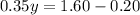
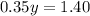
Divide both sides by 0.35.

`y=(1.40)/(0.35)`

`y=4`

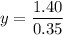
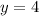
Substituting
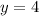`y=4` in (i), we get

`x=4+2`

`x=6`

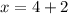
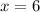
Therefore, the number of dimes is 6 and the number of quarters is 4.