Answer:

Explanation:
Given
![y = []x + 6](https://img.qammunity.org/2022/formulas/mathematics/high-school/1ziw2xt5i3dwxrv41tdmkq5al2wm9uu49z.png) --- equation 1
--- equation 1
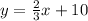 --- equation 2
--- equation 2
Required
Fill in the blank
A linear equation is represented as:

Where m represents the slope
If (1) and (2) are perpendicular, then:
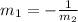
In
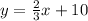 --- equation 2
--- equation 2
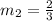
So, we have:
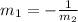
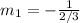
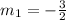
Hence, the complete equation is:
