Question

Given the function f(x) = 4x - 2, explain and show how to find the average rate of change between x = 2 and x = 4.

Answer 1

The average rate of change between x = 2 and x = 4 is of 4.

Explanation:

Average rate of change:

The average rate of change of a function f(x) in an interval [a,b] is given by:

`A = (f(b)-f(a))/(b-a)`

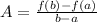
In this question:

`f(x) = 4x - 2, b = 4, a = 2`

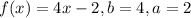
Thus:

`f(b) = f(4) = 4(4) - 2 = 16 - 2 = 14`

`f(a) = f(2) = 4(2) - 2 = 8 - 2 = 6`

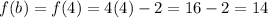
Average rate of change:

`A = (f(b)-f(a))/(b-a)`

`A = (14-6)/(4-2)`

`A = (8)/(2)`

`A = 4`

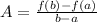
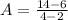
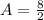
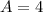
The average rate of change between x = 2 and x = 4 is of 4.