Question

For the given function, find the vertical and horizontal asymptote(s) (if there are any).

f(x) = (x-5)/(x^2-1)

Answer 1

The person above me has the answer LOL

Answer 2

See below for answers and explanations (as well as a graph attached)

Explanation:

The function
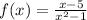`f(x)=(x-5)/(x^2-1)` can be written as
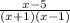`(x-5)/((x+1)(x-1))`, showing that there are 2 vertical asymptotes, which are at
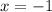`x=-1` and
`x=1` as they both make the denominator equal to 0.

Additionally, there would be a horizontal asymptote at
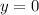`y=0` since the degree of the numerator is less than the degree of the denominator.

See the attached graph.