Question

Calculate the number of milliliters of 0.587 M NaOH required to precipitate all of the Fe3 ions in 197 mL of 0.654 M FeCl3 solution as Fe(OH)3. The equation for the reaction is: FeCl3(aq) 3NaOH(aq) Fe(OH)3(s) 3NaCl(aq)

Answer 1

Answer: The number of milliliters of 654 mL for 0.587 M NaOH required to precipitate all of the
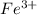`Fe^(3+)` ions in 197 mL of 0.654 M
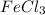`FeCl_(3)` solution as
`Fe(OH)_(3)`.

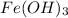Step-by-step explanation:

The reaction equation is as follows.

`FeCl_(3)(aq) + 3NaOH(aq) \rightarrow Fe(OH)_(3)(s) + 3NaCl(aq)`

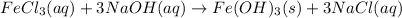
Therefore, moles of
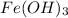`Fe(OH)_(3)` are calculated as follows.

Moles = Molarity of
`Fe(OH)_(3)`
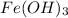
`*` Volume (in L)

= 0.654 M
`*` 0.197 L

= 0.128 mol

Now, according to the given balanced equation 1 mole of
`FeCl_(3)(aq)` reacts with 3 moles of NaOH(aq). Hence, moles of
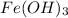`Fe(OH)_(3)` reacted are calculated as follows.

3
`*` 0.128 mol = 0.384 moles of NaOH

As moles of NaOH present are as follows.

Moles of NaOH = Molarity of NaOH
`*` Volume (in L)

0.384 mol = 0.587 M
`*` Volume (in L)

Volume (in L) = 0.654 L (1 L = 1000 mL) = 654 mL

Thus, we can conclude that the number of milliliters of 654 mL for 0.587 M NaOH required to precipitate all of the
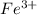`Fe^(3+)` ions in 197 mL of 0.654 M
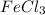`FeCl_(3)` solution as
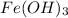`Fe(OH)_(3)`.