Question

The measure of arc XY is 85 degrees. The measure of arc WZ is 25 degrees. What is the measure of angle 3?

Answer 1

Answer: 30 degrees

Explanation:

need the points

Answer 2

Given:

Consider the below figure attached with this question.

To find:

The measure of angle 3.

Solution:

According to intersecting secant theorem, if two secants of a circle intersect each other outside the circle, then the angle on the intersection is half of the difference of the intercepted arc.

`\text{Angle on intersection}=(1)/(2)(\text{Major arc}-\text{Minor arc})`

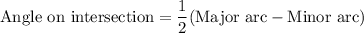
Using the intersecting secant theorem, we get

`m\angle 3=(1)/(2)(m(arcXY)-m(arcWZ))`

`m\angle 3=(1)/(2)(85^\circ-25^\circ)`

`m\angle 3=(1)/(2)(60^\circ)`

`m\angle 3=30^\circ`

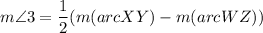
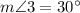
Therefore, the measure of angle 3 is 30 degrees.