Answer:
The margin of error is of 0.1282 = 12.82%.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.

In which
z is the z-score that has a p-value of
 .
.
The margin of error is of:

A survey conducted by General Motors of 38 drivers in America, 34 indicated that they would prefer a car with a sunroof over one without.
This means that
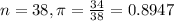
99% confidence level
So
 , z is the value of Z that has a p-value of
, z is the value of Z that has a p-value of
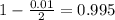 , so
, so
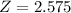 .
.
What is the margin of error?

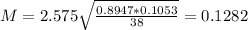
The margin of error is of 0.1282 = 12.82%.