1 Answer

Korun · Answer 1 · 2022-10-13T02:40:30+0000

Given:

Radius of a cylinder = 5 units.

Surface area of the cylinder =
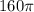
$160\pi$

To find:

The height of the right cylinder.

Solution:

Surface area of a cylinder is:

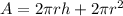
$A=2\pi rh+2\pi r^2$

Where, r is radius and h is the height of the cylinder.

Putting
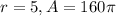$r=5, A=160\pi$ in the above formula, we get

$160\pi =2\pi (5)(h)+2\pi (5)^2$

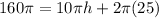
$160\pi =10\pi h+2\pi (25)$

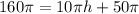
$160\pi =10\pi h+50\pi$

Subtract
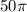$50\pi$ from both sides.

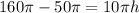
$160\pi -50\pi=10\pi h$

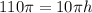
$110\pi=10\pi h$

Divide both sides by
$10\pi$ .

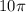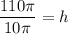
$(110\pi)/(10\pi)=h$

11=h

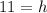
Therefore, the height of the cylinder is 11 units.

Please log in or register to add a comment.