Question

Find the derivative
f (x ) = (x-5)^2 (3-x)^2​

Answer 1

Given:

The function is

`f(x)=(x-5)^2(3-x)^2`

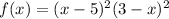
To find:

The derivative of the given function.

Solution:

Chain rule of differentiation:

`[f(g(x))]'=f'(g(x))g'(x)`

Product rule of differentiation:

`[f(x)g(x)]'=f(x)g'(x)+g(x)f'(x)`

We have,

`f(x)=(x-5)^2(3-x)^2`

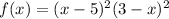
Differentiate with respect to x.

`f'(x)=(x-5)^2(d)/(dx)(3-x)^2+(3-x)^2(d)/(dx)(x-5)^2`

`f'(x)=(x-5)^2[2(3-x)(0-1)]+(3-x)^2[2(x-5)(1-0)]`

`f'(x)=(x^2-10x+25)(-6+2x)+(9-6x+x^2)(2x-10)`

`f'(x)=(x^2)(-6)+(-10x)(-6)+(25)(-6)+(x^2)(2x)-10x(2x)+25(2x)+(9)(2x)+(-6x)(2x)+x^2(2x)+9(-10)+(-6x)(-10)+x^2(-10)`

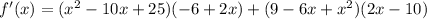
On further simplification, we get

`f'(x)=-6x^2+60x-150+2x^3-20x^2+50x+18x-12x^2+2x^3-90+60x-10x^2`

`f'(x)=(2x^3+2x^3)+(-6x^2-20x^2-12x^2-10x^2)+(60x+50x+18x+60x)+(-90-150)`

`f'(x)=4x^3-48x^2+188x-240`

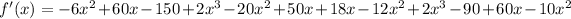
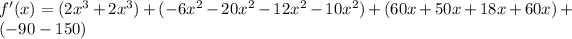
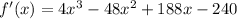
Therefore, the derivative of the given function is
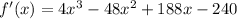`f'(x)=4x^3-48x^2+188x-240`.